Question

The midpoint of RT is S(0.5, –6.25). One endpoint is T(–5.5, 2.75). What are the coordinates of R? (–4.5, –15.25) (6.5, –9.75) (–4.5, –9.75) (6.5, –15.25)

Answer 1

The midpoint formula is this:
`( x_(midpt) , y_(midpt))=( ( x_(1) + x_(2) )/(2) , ( y_(1)+ y_(2) )/(2) )`. We have the coordinates of the midpoint and the coordinates of one of the endpoints, so our formula becomes:
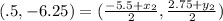
`(.5, -6.25)=( (-5.5+ x_(2) )/(2), (2.75+ y_(2) )/(2))`. Now group the x stuff together and solve for x2 and group the y stuff together and solve for y2, like this:
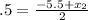
`.5= (-5.5+ x_(2) )/(2)`. Multiply both sides by 2 to get 1=-5.5+x2. Solve for x2 to get x2 = 6.5. Do the same with the y stuff:
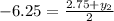
`-6.25= (2.75+ y_(2) )/(2)`. Multiply both sides by 2 to get-12.5=2.75+y2 and solving for y2 gives you -15.25. So your answer is the last of your choices above. Phew!

Answer 2

D, (6.5, -15.25) , JUST GOT IT CORRECT