Let's set variables to the different tickets:
S is senior tickets, and A is adults.
If there were 414 tickets sold, then
414 = A + S
If the total amount sold was $6173 and adults tickets were $20 and senior tickets were $13, then
6173 = 20A + 13S
We can then put these two equations into a system of equations:
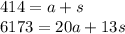
We can single out one of the variables in the simpler equation to solve for the other:
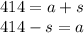
Now that we have A, we can plug it into the other equation:
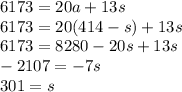
We plug in 414 - S for A, then we factor in the 20. Then we subtract 8280 from both sides and combine the two Ss. Then we divide both sides by -7.
The theatre sold 301 senior tickets and 113 adult tickets.