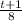(a)
The inverse is when you swap the variables and solve for y.
g(t) = 2t - 1 (Note: g(t) represents y)
rewrite as: y = 2t - 1
swap the variables: t = 2y - 1
solve for y: t + 1 = 2y
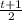
= y
Answer for (a):
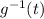 =
=
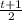
(b)
Same steps as part (a) above:
h(t) = 4t + 3
rewrite as: y = 4t + 3
swap the variables: t = 4y + 3
solve for y:
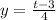 Answer for (b):
Answer for (b):
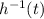 =
=
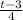
(c)
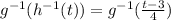
replace all t's in the
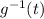
equation with
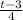
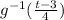
=
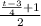
=
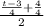
=
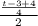
=
 Answer for (c):
Answer for (c):
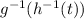 =
=
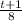
(d)
h(g(t)) = h(2t - 1) = 4(2t - 1) + 3 = 8t - 4 + 3 = 8t - 1
Answer for (d): h(g(t)) = 8t - 1
(e)
h(g(t)) = 8t - 1
y = 8 t - 1
t = 8y - 1
t + 1 = 8y
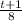
= y
Answer for (e): inverse of h(g(t)) =