Question

Henry constructed Circle a with a radius of 10 units. He then created a sector as shown in the figure below period which of the following expressions would help him find the area of the shaded sector

Answer 1

b. 240/360 (100pi)

1. 120°/360° = x/100pi (the area of the circle)
2. cross multiply
3. find an equal expression

Answer 2

`(120^(\circ))/(360^(\circ)) * 100\pi`

Explanation:

Radius of Circle : 10 units

Measure of angle of given sector = 120°

Formula of area of sector =
`(\theta)/(360^(\circ)) * \pi r^2`

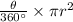
Substitute the values in the formula .

Area of sector =
`(120^(\circ))/(360^(\circ)) * \pi (10)^2`

=
`(120^(\circ))/(360^(\circ)) * 100\pi`

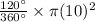
So, Option 2 is correct.

Hence the expression would help him find the area of the shaded sector is
`(120^(\circ))/(360^(\circ)) * 100\pi`