Question

Find an equation in standard form for the hyperbola with vertices at (0, ±6) and asymptotes at y = ±3 divided by 2.x

Answer 1

The equation of hyperbola with vertices at (0,±6) and asypmtotes at
`y= ±(3)/(2) x`

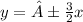
Explanation:

Given

vertices of hyperbola at (0,±6)

It means hyperbola along y axis and centre at (0,0)

Asymptotes
`y=±(3)/(2)`

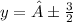
We know that equation of hyperbola along y axis

`(y^2)/(a^2) -(x^2)/(b^2) =1`

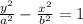
a=6

We know that the general equation of hyperbola asymptotes and vertcal transverse axis

y=
`±(a)/(b) x`

`(3)/(2) x`=
`(6)/(b) x`

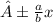
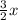Both side cancel x we get

`b=(6*2)/(3)`

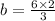
b=4

When we take
}{b}x[/tex]

Then we get b=4

Now, put the value of a and b in the equation of hyperbola we get

`(y^2)/(6^2) -(x^2)/(4^2)`=1

`(y^2)/(36) -(x^2)/(16) =1`

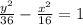
Hence, the required standard equation of hyperbola

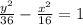`(y^2)/(36) -(x^2)/(16) =1`.

Answer 2

The standar form for the hyperbola is:

(y^2/a^2)-(x^2/b^2)=1

a=6
a^2=36

The equation of the asymptotes is:

y=±(a/b)x

a/b=3/2
b=4
b^2=16

Therefore, the equation is:

(y^2/a^2)-(x^2/b^2)=1
(y^2/36)-(x^2/16)=1