Question

a kite string is 220 feet long from the kite to the ground. the string makes a 45 degree angle with the ground. about how high off the ground is the kite?

Answer 1

The kite is approximately 155.6 feet off the ground.

Step-by-step explanation:

To find how high off the ground the kite is, we can use trigonometry. Since the string makes a 45 degree angle with the ground, we can use the sine function to find the height. The sine of 45 degrees is equal to the opposite side (height) divided by the hypotenuse (string length), so we can set up the equation: sin(45°) = height/220. Solving for the height, we get: height = sin(45°) * 220. Using a calculator, we find that sin(45°) is approximately 0.7071. Therefore, the height of the kite is approximately 0.7071 * 220 = 155.6 feet.

Answer 2

The best way to solve these types of questions is to draw a diagram. In this case, we can draw a right triangle with the kite string as the hypotenuse. Since a right triangle has a right angle, and the other angle is 45 degrees, we can deduce that the last angle is also 45 degrees because the sum of all angles in any triangle is equal to 180.

There is a special ratio with right angles that have the angles 45, 45, and 90. The ratio tells us that the hypotenuse is
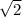
`√(2)` times as large as the sides.

220 = x
`√(2)`
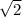
220
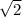
`√(2)`/2 = x
110
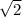
`√(2)` = x

The kite is 110
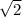
`√(2)` ft. from the ground.