Question

Find the sum of the following infinite geometric series, if it exists.

60 + 84 + 117.6 + 164.64 +…



Does not exist



1456



2467



3672

Answer 1

`q=(84)/(60)=1.4 > 1\ \text{ therefore you answer: Does not exist}`

Answer 2

Answer: Does not exist

Explanation:

The given geometric series :
`60 + 84 + 117.6 + 164.64 +…`

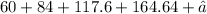
Here the first term :
`a=60`

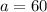
The second term :
`ar=84`

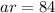
Now, the common ratio in this series will be :_

`r=(ar)/(a)=(84)/(60)=1.4>1`

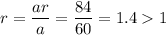
We know that if the common ratio in infinite geometric series does not lies between (-1,1) , then the series is diverging. And the sum of the infinite geometric series will not exists.

Hence, the sum of the given infinite geometric series does not exist.