We'll say that months = n.
Make a set of the DVD's sold:
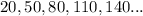
In month 1, Aaron sold 20 DVDs. There is no data for month 0.
There is a constant increase of 30 DVDs every month. We can make an equation out of this to fit this data set:
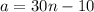
a represents the DVDs made.
We need to subtract 10 in this equation, as the starting point is 20, and the increase of 30 is different from the increase from n = 0 to n = 1.
We are looking for the amount of DVDs Aaron sold on the 13th month. Plug 13 into the equation:

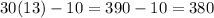
The predicted number of DVDs Aaron will sell on the 13th month is
380.