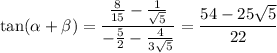Recall some identities:
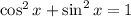
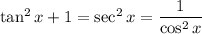
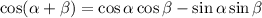

Not sure what part (c) is asking for, but I assume it's

, in which case

If
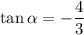
, then
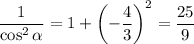
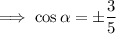
We know that

lies in quadrant 2, i.e.
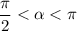
, so we expect

. So we take the negative root. We also find that
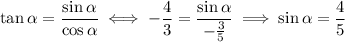
If
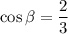
, then

Since
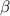
lies in quadrant 1, i.e.
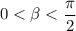
, we know that
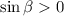
, so we take the positive root.
Now,
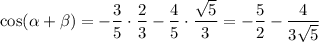
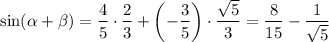
Then it follows that