Question

Please help with this vertical asymptote of rational functions problem

A. x= -5
B. x=0
C. x= -2
D. x= 5

Answer 1

The answer is the first option, which is:

x=-5

The explanation is shown below:

1.To solve this problem you must apply the proccedure shown below:

2. You must factor the denominator, as following:

f(x)=(5x+10)/(x^2+7x+10)
f((x)=(5x+10)/(x+2)(x+5)

x+5=0
x=-5

Answer 2

The expression can be written in simplified form as:


`f(x)= (5x+10)/( x^(2) +7x+10) \\ \\ f(x)= (5(x+2))/( x^(2) +2x+5x+10) \\ \\ f(x)= (5(x+2))/((x+2)(x+5))`

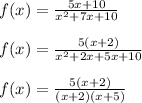
x+2 occurs in both numerator and denominator. This means there is a hole at x=-2, this can also be seen from the graph.

So, the vertical asymtptote will occur at the point where:
x+5=0
⇒
x = -5

So, option A gives the correct equation of the vertical asymptote of the function