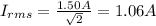Question

You have a special light bulb with a very delicate wire filament. the wire will break if the current in it ever exceeds 1.50 a, even for an instant. what is the largest root-mean-square current you can run through this bulb? 31.2 . a sinusoidal current

Answer 1

The root mean square of the current is given by

`I_(rms) = (I_0)/( √(2) )`
where
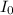
`I_0` is the maximum value of the current.

In our problem, the maximum current allowed without breaking the filament is equal to
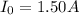
`I_0=1.50 A`. Therefore, the largest root-mean-square current allowed without breaking the wire is

`I_(rms)= (1.50 A)/( √(2) )=1.06 A`