Question

suppose a triangle has two sides of length 2 and 5 and that the angle between these two sides is 60 degrees. What is the length of the third side of the triangle? 5 , 2sq root 3, sq root 3, sq root 19

Answer 1

square root of 19 is the answer

Answer 2

Answer:
`c=√(19)`

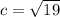
Explanation

Two sides and included angle is known to us .cosine rule can be applied to find the third side.

Let a =2 ,b=5 and <C=60 degrees ,we need to find the third side c.

According to cosine rule :

`c^(2) =a^(2) +b^(2) -2abcos C.`

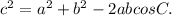
Substituting the values we have:

`c^(2)=2^(2) +5^(2) -2(2)(5)cos 60`

`c^(2) =4+25-20 ((1)/(2) )`

`c^(2) =29-10=19`

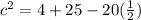
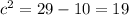
Taking root of both sides

`c=√(19)`

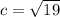
The last option is correct.