Question

What is the solution of the system? use elimination . -12x-y=6 17x+y=4

Answer 1

To clarify the systems of equations:


`-12x - y = 6`

`17x + y = 4`

We already have opposite coefficients for y, so combine the two equations together:


`5x = 10`

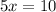
Divide both sides by 5 to get x by itself:


`x = 2`

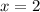
Because we now know x, we can plug it into one of the equations.

We will plug this value into the first equation:


`-12(2) - y = 6`


`-24 - y = 6`

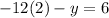
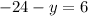
Add 24 to both sides.


`-y = 30`

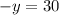
Divide both sides by -1 to get y by itself.


`y = -30`

Using elimination, x = 2 and y = -30.