The equation of a circle is:
(x - h)² + (y - k)² = radius²
where (h, k) is the center.
We can plug in the given information from the description and solve for the radius.
(x - 2)² + (y - 4)² = radius²
To find the radius, we do the distance formula from the center to the point on the circle.
Distance =

D =
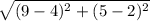
D =
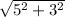
D =
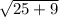
D =
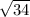
That is your radius. Now we plug it in.
(x - 2)² + (y - 4)² = (√34)²
Your final answer is:
(x - 2)² + (y - 4)² = 34