Question

The rectangle below has an area of x^2-4x-12 square meters and a length of x+2what expression represents the width of the rectangle?

Answer 1

x^2 - 4x - 12
x - 6
x +2

If L equals to x + 2 than W will equal to x - 6

Answer 2

The width is x-6.

Explanation:

Givens:

• Area of the rectangle
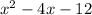  `x^2-4x-12` square meters.
• The length is
  `x+2`

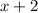
We know that, the are of a rectangle is:

`A=(length)(width)`

`x^2-4x-12=(x+2)w`

`(x^2-4x-12)/(x+2)=w`

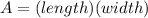
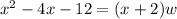
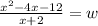
Now, to solve this, we factorize the numerator.

`x^2-4x-12=(x-a)(x+b)`

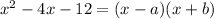
So, we need to find two number which product is 12 and difference is 4.

`x^2-4x-12=(x-6)(x+2)`

Now, we replace this result in the fraction expression:

`(x^2-4x-12)/(x+2)=w\\w=((x-6)(x+2))/((x+2))\\w=x-6`

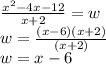
Therefore, the width is x-6.