Question

In a right rectangular pyramid with base edges a=18 cm and b=10 cm the slant height towards a is k=13 cm while the slant height towards b is l=15 cm. Find the surface area of a prism

Answer 1

The surface area of a pyramid is 564 cm².

Explanation:

It is given that the rectangular base of the pyramid has dimensions 18 cm and 10 cm. The slant height towards a is k=13 cm while the slant height towards b is l=15 cm.

Area of a rectangle is

`A=length* width`

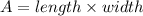
The area of base is

`A_1=18* 10=180`

The area of a triangle is

`A=(1)/(2)* base * height`

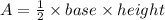
Area of a triangle with base 18 and height 13 is

`A_2=(1)/(2)* 18 * 13=117`

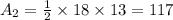
Area of a triangle with base 10 and height 15 is

`A_3=(1)/(2)* 10 * 15=75`

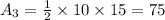
Total surface area of a pyramid is

`A_1+2(A_2)+2(A_3)=180+2(117)+2(75)=564`

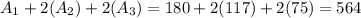
Therefore the surface area of a pyramid is 564 cm².

Answer 2

Surface Area of pyramid=(sum of four isosceles triangle)+ rectangular base
=(a*k+b*I) + a*b
=(18*13+10*15)+ 18*10
=564 cm²