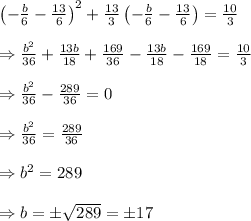Given that the difference between the roots of the equation
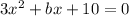
is
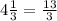
.
Recall that the sum of roots of a quadratic equation is given by
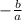
.
Let the two roots of the equation be

and
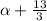
, then
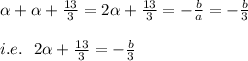
. . . (1)
Also recall that the product of the two roots of a quadratic equation is given by
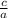
, thus:
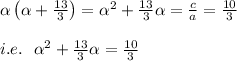
. . . (2)
From (1), we have:
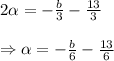
Substituting for alpha into (2), gives: