Question

F(x)=x^2-x-1 What is the average rate of change of f over the interval -1<=x<=1

Answer 1

In calculus, we use derivatives to find the instantaneous rate of change at any point on a graph. To find the average rate of change, we just find the slope of the secant line that intercepts two points on the graph.

We find slope with the following equation:


`m = (y_1 - y_2)/(x_1-x_2)`

In this case, we are looking for the slope from x = -1 to x = 1. We have both x values, so next we need the y values.

F(-1) = (-1)^2 - (-1) - 1 = 1

F(1) = (1)^2 - (1) - 1 = -1

Now plug in the x and y values to find the slope:


`(1-(-1))/(-1-1) =-1`

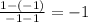The answer is -1.