Question

A ball is thrown upward from the top of a building. The function below shows the height of the ball in relation to sea level, f(t), in feet, at different times, t, in seconds: f(t) = −16t2 + 34t + 546 The average rate of change of f(t) from t = 5 seconds to t = 7 seconds is _____ feet per second.

Answer 1

-158

Explanation:

First we evaluate the function for t = 5 and t = 7:

f(5) = -16(5²)+34(5)+546

= -16(25)+170+546

= -400+170+546 = 316

f(7) = -16(7²)+34(7)+546

= -16(49)+238+546

= 0

The average rate of change is found using the formula

`m=(y_2-y_1)/(x_2-x_1)`

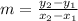
Using our two values of the function as y and the times, t, as x, we have:

y = (0-316)/(7-5) = -316/2 = -158

Answer 2

`\bf slope = m = \cfrac{rise}{run} \implies \cfrac{ f(x_2) - f(x_1)}{ x_2 - x_1}\impliedby \begin{array}{llll} average~rate\\ of~change \end{array}\\\\ -------------------------------\\\\ f(x)= -16t^2+34t+546 \qquad \begin{cases} x_1=5\\ x_2=7 \end{cases}\implies \cfrac{f(7)-f(5)}{7-5} \\\\\\ \cfrac{[-16(7)^2+34(7)+546]~~-~~[-16(5)^2+34(5)+546]}{2} \\\\\\ \cfrac{[0]~~-~~[316]}{2}\implies \cfrac{-316}{2}\implies \cfrac{-158}{1}\implies -158`