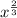Answer : The correct option is,
Step-by-step explanation :
The given expression is :
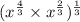
First we have to solve the term present in the bracket.
Identity used :
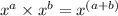

By the adding the powers of 'x', we get:
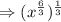
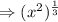
Now we have to use identity
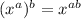 , we get :
, we get :
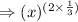
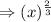
Therefore, the correct option is,