Question

when ashley buys 7 postage stamp booklets she gets 140 stamps.she get 60 stamps in three booklets. write an equation to find the number of postage stamps, p , she gets in b booklets

Answer 1

We can put this information into two equations:

7 booklets = 140 stamps
3 booklets = 60 stamps

Let us then focus on one equation to find the relationship:

7 booklets = 140 stamps

If we put that into a fraction, we get
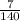
`(7)/(140)` or
`(1)/(20)`.

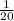
This means that for every booklet, Ashley gets 20 stamps; or rather, each booklet has 20 stamps.

We can then see that this relationship holds true for the second equation as well:


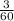
`(3)/(60)` or
`(1)/(20)`.

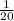
To write a general equation for this relationship using p for the number of postage stamps, and b for the number of booklets:

p = 20b

Where b is equal to p.