Answer:
Hence, the sum using summation notation, assuming the suggested pattern continues
 is:
is:
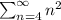
Explanation:
We have to write the sum using summation notation, assuming the suggested pattern continues:

Clearly we may also write this pattern as:
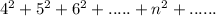
So, in terms of the summand it is written as:
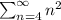
( We have started our summation from 4 since the term in the summation starts with 16 which is 4^2 and goes to infinity )