Question

Determina si 2018 es un termino de la siguiente progresion aritmetica 17,46,75,104,133,....

Answer 1

2018 is the 70th term of the progression.

Step-by-step explanation
We start out finding the common difference of the progression:
46-17 = 29

Now we write the explicit formula for the sequence. It is of the form

`a_n=a_1+d(n-1) \\ a_n=17+29(n-1)`

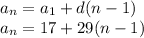
We set this equal to 2018 to see if the answer is a whole number. If it is, it will be the term number that gives us 2018:

2018=17+29(n-1)

Using the distributive property,
2018=17+29*n-29*1
2018=17+29n-29

Combine like terms:
2018=29n-12

Add 12 to both sides:
2018+12=29n-12+12
2030=29n

Divide both sides by 29:
2030/29=29n/29
70=n

Since n=70, this means 2018 is the 70th term of the sequence.