Answer:
x=+4, x= -4
Explanation:
the solutions to the quadratic equation
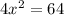
To solve the quadratic equation , solve the equation for x
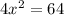
Divide both sides by 4
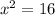
To eliminate square from x , take square root on both sides
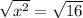
When we take square root we put +-
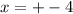
x=+4, x= -4