To solve this, we are going to use the present value formula:
![PV=P[ (1-(1+ (r)/(n))^(-kt) )/( (r)/(n) ) ]](https://img.qammunity.org/2019/formulas/mathematics/college/ix7ttvafv7z10uh2xovkvslovcngb8c98v.png)
where
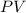
is the present value
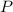
is the periodic payment

is the number of times the interest is compounded per year

is the number of payments per year

is the number of years
We know for our problem that Xavier deposits $6 daily into an interest bearing account, so

and
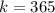
. To convert the interest rate to decimal form, we are going to divide the rate by 100%
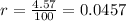
Since the interest is compounded annually, it is compounded 1 time per year; therefore,
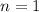
. We also know that Xavier renovates in five years, so
Lets replace the values in our formula:
![PV=P[ (1-(1+ (r)/(n))^(-kt) )/( (r)/(n) ) ]](https://img.qammunity.org/2019/formulas/mathematics/college/ix7ttvafv7z10uh2xovkvslovcngb8c98v.png)
![PV=6[ (1-(1+ (0.0457)/(1))^(-(365)(5)) )/( (0.0457)/(1) ) ]](https://img.qammunity.org/2019/formulas/mathematics/college/a472elbmqutbwbjfgwd2l6ly5akgsq06jw.png)
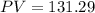
We can conclude that the present value of Xavier's investment is
$131.29