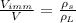Question

What does Archimedes' principle say about how the buoyant force relates to the fluid involved?

Answer 1

The buoyant force is equal to the weight of the volume of fluid displaced by the solid immersed into it, and it is equal to:

`B=\rho_L V_(imm) g`
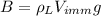
where

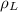
`\rho_L` is the density of the fluid

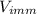
`V_(imm)` is the volume of the part of the solid immersed in the fluid
g is the gravitational acceleration

We see that the greater the density of the fluid, the greater the buoyant force that pushes the object upward. When the object floats in the fluid, the buoyant force is equal to the weight of the object, mg:

`mg=\rho_L V_(imm) g` (1)
and since the mass of the solid is equal to the product between its density and its volume:
`m=d_s V`
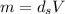
we can rewrite (1) as

`\rho_S V =\rho_L V_(imm)`
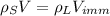
where
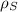
`\rho_S` is the density of the object and
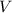
`V` is its total volume. So the fraction of the object immersed in the fluid is

`(V_(imm))/(V)= (\rho_s)/(\rho_L)`