Answer:
Zero real solutions does the system have.
Explanation:
Given the system of equations:
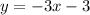

Equate these equations we have;
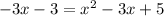
Add 3x to both sides we have;
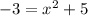
Subtract 5 from both sides we have;
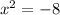
⇒
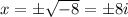
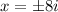 , where i is the imaginary part.
, where i is the imaginary part.
both solution are imaginary.
Therefore, the system of equation does not have any real solutions.