Polynomial equations that can be solved with the quadratic formula have the following properties, assuming all like terms have been simplified.
1. degree = 2 (i.e. the highest power equals exactly two)
2. the linear term (e.g. 4x, or -5x...) and constant term (e.g. 5, -30, pi, etc.) may or may not be present.
Now let's simplify and examine the given equations, and see if each can be solved with the quadratic formula:
A.
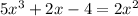
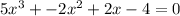
The degree (highest power) is three, so it is not "exactly two".
NO.
B.
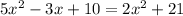

The degree (highest power) is two, so it is "exactly two".
YES.
C.
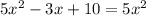
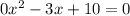
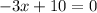
The degree (highest power) is one, so it is not "exactly two".
NO.
D.
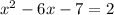
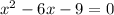
The degree (highest power) is two, so it is "exactly two".
YES.
Note that it is very important to simplify the equations before checking the degree.
If you need further explanations, please feel free to post in comments.