Question

A music buff wants to estimate the percentage of students at a midwestern university who believe that elvis is still alive. how many students should he include in a random sample if he wants a 90% confidence interval that is less than 10 percentage points wide? (choose the sample size that is closest to your solution) question 7 options:

Answer 1

Since we are not given any information about the proportion, we will assume the sample proportion to be 0.50

so,
p = 0.50
The Error is 10% percentage point. This means that on either side of the population proportion the error is 5% so E = 0.05
z = 1.645 (Z value for 90% confidence interval)

The margin of error for population proportion is calculated as:


`E=z* \sqrt{ (p(1-p))/(n) } \\ \\ (E)/(z) = \sqrt{ (p(1-p))/(n) } \\ \\ ( (E)/(z) )^(2)= (p(1-p))/(n) \\ \\ n= p(1-p)* ((z)/(E))^(2) \\ \\ n=271`

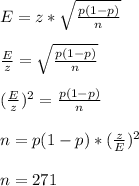This means 271 students should be included in the sample