We assume you want to find values of x that make the expression true.
Use the rules of logarithms to rewrite as a single log. Then take the antilog and solve the resulting quadratic.

The term
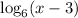
is only defined for x > 3, so the only solution to this equation is
x = 4.