Question

The cylinders are similar the volume of the larger cylinder is 1600 cubic centimeters what is the volume of the smaller cylinder

Answer 1

We are given Volume of the larger cylinder is = 1600 cubic centimeters.

Height of the larger cylinder = 16 cm.

We know formula of volume of a cylinder V=
`\pi r^2h`, where r is the radius of cylinder, h is the height of the cylinder.

Plugging value of V and h of larger cylinder, we get

`1600=\pi r^2(16).`

Dividing both sides by 16, we get

`(1600)/(16)=(\pi r^2 (16))/(16)`

`r^2=100`

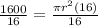
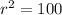
Taking square root on both sides, we get

r=10.

Therefore, radius of the larger cylinder is 10 cm.

We are given cylinders are similar .

Note: The radii and heights of similiar cylinders are in same ratio.

Therefore, we can setup a proportion:

Let us take radius of small cylinder is x.

`(x)/(10)=(4)/(16)`

`(x)/(10)=(1)/(4)`

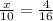
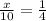
Multiplying both sides by 10, we get

`10 * (x)/(10)=10 *(1)/(4)`

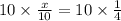
x=2.50.

Therefore, radius of the small cylinder = 2.5 cm.

Now, plugging radius =2.5 and height = 4 in the formula of volume the cylinder, we get

`V=\pi (2.5)^2(4)=\pi (6.25)(4) =25 \pi \ cm^3.`

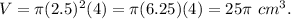
Therefore, correct option is 25 pi cm^3.