Question

Can someone explain to me how to find the area of this kite? i understand the normal formula and everything, just not this problem. thanks!

Answer 1

First, I'll clarify what the line and double-lines in some of the triangle's sides mean.

The two sides with one perpendicular line, means the two sides are equal. The two sides of the sides with two perpendicular lines, means that two sides are equal.

We have four right triangles, we'll start by focusing in the two right triangles in our right. This two triangles have a hypotenuse of 18, a side of 16, and an unknown side (which is shared by the other two right triangles in the left).

We calculate this unknown side by using the Pythagorean Theorem. Let
`x` be the unknown side of the two right triangles on the left, then:


`18^2=16^2+x^2`⇒

`x= √(18^2-16^2)= √(68)=8.24`

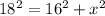
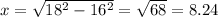
Now, lets focus in the two right triangles in the left. This two triangles have a hypotenuse of 11, a side of 8.24, and an unknown side.
Lets call this unknown side
`y` and solve for it by using the Pythagorean Theorem:


`y= √(11^2-8.24^2)= √(53.10)=7.29`

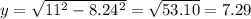
Now we know all sides of the four right triangles that make up the kite.
To get the total area of the kite, we get the area of the four right triangles and add them:


`(7.29+8.24)/(2)+(7.29+8.24)/(2)+ (16+8.24)/(2)+(16+8.24)/(2)`

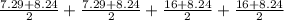So, the are is equal to 39.77