Question

The number of potholes in any given 1 mile stretch of freeway pavement in Pennsylvania has a bell-shaped distribution. This distribution has a mean of 42 and a standard deviation of 10. Using the empirical (68-95-99.7) rule, what is the approximate percentage of 1-mile long roadways with potholes numbering between 22 and 52

Answer 1

81.5% of 1-mile long roadways with potholes numbering between 22 and 52

Explanation:

The Empirical Rule states that, for a normally distributed random variable:

68% of the measures are within 1 standard deviation of the mean.

95% of the measures are within 2 standard deviation of the mean.

99.7% of the measures are within 3 standard deviations of the mean.

In this problem, we have that:

Mean of 42, standard deviation of 10.

The normal distribution is symmetric, so 50% of the measures are below the mean and 50% are above the mean

Approximate percentage of 1-mile long roadways with potholes numbering between 22 and 52:

22 = 42 - 2*10

So 22 is two standard deviations below the mean. Of the 50% of the measures that are below the mean, 95% are between two standard deviations below the mean(22) and the mean(42).

52 = 42 + 10

So 42 is one standard deviation above the mean. Of the 50% of the measures that are above the mean, 60% are between the mean(42) and one standard deviation above the mean(52).

In the desired interval, the percentage is:

`P = 0.5*0.95 + 0.5*0.68 = 0.815`

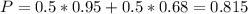
81.5% of 1-mile long roadways with potholes numbering between 22 and 52