Question

In △QRS, QR = 7, RS = 11, and m∠R = 42. In △UVT, VT = 28, TU = 44 and m∠T = 42. Are the polygons similar? If they are, write a similarity statement and give the similarity ratio

A) QRS = TUV; 11:28
B ) QRS =TUV; 7:44
C) The triangles are not similar
D) QRS~VTU; 1:4

Answer 1

D) Δ QRS ~ Δ VTU; 1:4

Explanation:

Given,

In triangle QRS,

QR = 7, RS = 11, and m∠R = 42,

In triangle UVT,

VT = 28, TU = 44 and m∠T = 42

Since,

`(QR)/(VT)=(7)/(28)=(1)/(4)`

`(RS)/(TU)=(11)/(44)=(1)/(4)`

`\implies (QR)/(VT)=(RS)/(TU)`

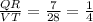
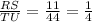
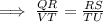
Also, m∠R = m∠T ⇒ ∠R ≅ ∠T

Hence, by SAS similarity postulate,

`\triangle QRS\sim \triangle VTU`

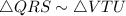
And, their similarity ratio is 1 : 4,

⇒ Option D is correct.

Answer 2

Since we are going to check the similarity of the triangles and we have given two sides and angle we have to check the Side-Angle-Side option of the similarity. We have equal angles. Then, we have to check the proportionality of the sides. If these triangles are equal, then VT/QR=UT/RS. If we calculate it, 28/7=44/11=4. This last number is the similarity ratio and since we have proportionality, ΔQRS ~ ΔVTU. The correct answer is D)