Question

Suppose a normal distribution has a mean of 222 and a standard deviation of 16. What is the probability that a data value is between 190 and 230? Round your answer to the nearest tenth of a percent. A. 91.0% B. 66.9% C. 53.3% D. 84.0%

Answer 1

Answer: B. 66.9%

A P E X

Suppose a normal distribution has a mean of 222 and a standard deviation of 16. What is the probability that a data value is between 190 and 230? Round your answer to the nearest tenth of a percent. A. 91.0% B. 66.9% C. 53.3% D. 84.0%

Answer 2

Mean of the distribution = u = 222
Standard Deviation = s = 16

We have to find the probability that a value lies between 190 and 230.

First we need to convert these data values to z score.


`z= (x-u)/(s)`

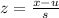
For x = 190,

`z= (190-222)/(16)=-2`

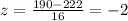
For x = 230

`z= (230-222)/(16)=0.5`

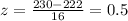
So, we have to find the percentage of values lying between z score of -2 and 0.5

P( -2 < z < 0.5) = P(0.5) - P(-2)

From standard z table, we can find and use these values.

P(-2 < x < 0.5 ) = 0.6915 - 0.0228 = 0.6687

Thus, there is 0.6887 probability that the data value will lie between 190 and 230 for the given distribution.