This is a problem of permutation. In mathematics, the permutation relates to the fact of arranging all the member of a set into order. So, the k permutations of n are the different ordered arrangements of a k element subset of a n set. In the problem above k = 9 are the elements which are all the numerals available. On the other hand, n set is equal to 4. So, the formula of such k-permutations of n is given by:
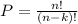
Solving for k = 9 and n = 4:
