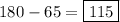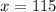We know the sum of all angles in a triangle is
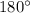
and they have given both the triangles two of the angles.
*****
For the one on the right, we have

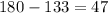
The bottom left angle of the triangle on the right is
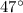
.
*****
For the triangle on the left, we do the same process.
The bottom right angle of the triangle on the left is
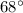
.
*****
Now we can find the last angle of the smaller triangle created by the overlapping larger triangles.
Using the same method as the previous ones, we get
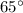
.
*****
A straight angle is
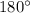
.