Question

When finding the margin of error for the mean of a normally distributed population from a sample, what is (photo below) , assuming a confidence level of 74%?

A.) 0.13
B.) 0.26
C.) 0.74
D.) 0.87

Answer 1

The value of significance level or α = 0.26

Explanation:

Confidence level = 74%

To calculate : α which is called significance level

The significance level α is defined as the probability of making the wrong decision when the null hypothesis is given to be true.

This significance level is used in the hypothesis tests and in calculating the critical value and margin of error.

Symbolically, to find the value of significance level or the value of α we simply subtract the given confidence level in the decimal form from 1

`\text{Significance Level, }\alpha=1-\frac{\text{Confidence Level}}{100}\\\\\implies\alpha=1-(74)/(100)\\\\\implies\alpha=1-0.74\\\\\bf\implies\alpha=0.26`

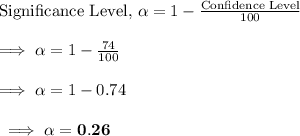
Hence, the value of significance level or α = 0.26

Therefore, the correct answer is B.) 0.26

Answer 2

`\boxed{\boxed{\alpha=0.26}}`

Explanation:

The significance level α is the probability of making the wrong decision when the null hypothesis is true. Alpha levels which is also called as significance levels are used in hypothesis tests.

Mathematically, the value of α is determined by subtracting the level of confidence from 1, and then writing the result as a decimal.

As the confidence level is given as 74%, so the significance level would be,

`=1-\alpha`

`=1-0.74`

`=0.26`