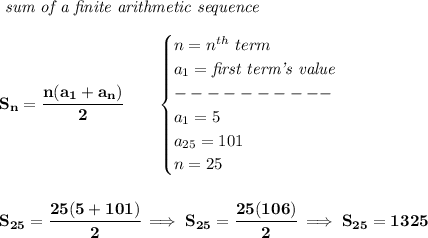
so they start off with 5 bricks, then they add 2 bricks on the left side and 2 bricks on the right side, namely 4 bricks, so the first row is 5 bricks, the next row is 5+4 or 9 bricks and so on.
5, 9, 13, 17.... <--- as you can see the "common difference" is 4.