Question

Which quadratic equation equation is equivalent to (x^2-1)^2 -11(^2-1) +24=0

Answer 1

`(x^2-1)^2-11(x^2-1)+24=0`

Use substitution:
`x^2-1=t`


`t^2-11t+24=0\\\\t^2-8t-3t+24=0\\\\t(t-8)-3(t-8)=0\\\\(t-8)(t-3)=0\iff t-8=0\ \vee\ t-3=0\\\\t=8\ \vee\ t=3`

we're going back to substitution:


`x^2-1=8\ \vee\ x^2-1=3\ \ \ \ |add\ 1\ to\ both\ sides\ of\ the\ equations\\\\x^2=9\ \vee\ x^2=4`

therefore


`x=\pm\sqrt9\ \vee\ x=\pm\sqrt4\\\\\boxed{x=-3\ \vee\ x=3\ \vee\ x=-2\ \vee\ x=2}`

Answer 2

x = ± 3 and ± 2

Explanation:

The given quadratic equation is

`(x^(2)-1)^(2)-11(x^(2)-1)+24=0`

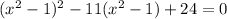
To make this question easier we will consume ( x² -1 ) = a

a² - 11a + 24 = 0

Now we can factorize this equation easily

a²- 8a - 3a + 24 = 0

a (a-8) - 3 ( a-8) = 0

( a-3 ) ( a-8 ) = 0

Therefore, a - 3 = 0 ⇒ a = 3

or a - 8 = 0 ⇒ a = 8

Now we put the value a

when a = 3 (x² - 1) = 3

x² = 3 + 1 = 4

x = √4

= ± 2

when a = 8 (x² - 1) = 8

x² = 9

x = √9

= ± 3

Therefore, x = ± 3 and ± 2 will be the answer.