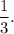Answer: The required probability that the selected egg is a cracked brown egg is
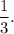
Step-by-step explanation: Given that a basket is filled with 7 white eggs and 14 brown eggs and half of the brown eggs are cracked. An egg is randomly selected from the basket.
We are to find the probability that the selected egg is a cracked brown egg.
Since half of the brown eggs are cracked, so the number of cracked brown eggs will be
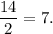
Let S denote the sample space for the experiment of selecting an egg from the basket and A denote the event that the selected egg is a cracked brown egg.
Then,
n(S) = 7 + 14 =21
and
n(A) = 7.
Therefore, the probability of event A is given by
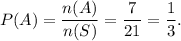
Thus, the required probability that the selected egg is a cracked brown egg is