Question

What are the zeroes of y = x^2 – 4x – 5?

Answer 1

Start by factoring
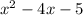
`x^2 - 4x - 5`. Your two factors will be in the form (x + a) and (x + b). You want two numbers a and b to multiply to -5 and add to -4. Test out various numbers, and you'll find that -5 and 1 work!

That means your factors are (x - 5) and (x + 1). You can double check this by foiling and seeing if you get your original equation.

Since you want to find the zeroes for that equation, that means y = 0. Remember that anything multiplied by 0 is 0, so you can set each of your factors equal to zero and solve for x. The x-values are your zeros:
x - 5 = 0
x = 5

x + 1 = 0
x = -1

-----

Answer: 5 and -1

Answer 2

x=5 or x=-1

Explanation:

The zeroes of the equation is when the x values when y=0.

We can determine this using a method that when we times out the brackets of our simplification we arrive at the original equation. We know that we must get -5 and -4x and x^2:

We can write:

`y=x^2-4*x-5=0`

`(x-5)*(x+1)=0`

If we use the rules of algebra and multiply each term with each other:

`x*x+x*1-5*x-5*1=0`

We can simplify:

`x^2-4*x-5=0`

We end up with the original equation:

Therefore the zeros of the equation is when:

`(x-5)*(x+1)=0`

`x-5=0` and
`x+1=0`

`x=5`

[tex]x=-1[/tex[

x=5 or x=-1