Question

AaBbCc x AaBbCc (use for all 3 questions) 1. What is the probability that this individual will: AABBcc 2. What is the probability that this individual will: AaBBcc 3. What is the probability that this individual will: AaBbCc

Answer 1

Answer: 1. P = 1/64

2. P = 1/32

3. P = 1/8

Explanation:

In genetics, an ofspring inherits one copy of an allele of each parent, which can be described in the tables below called Punnett Square:

For crossing Aa x Aa:

A a

A AA Aa

a Aa aa

For crossing Bb x Bb:

B b

B BB Bb

b Bb bb

For crossing Cc x Cc:

C c

C CC Cc

c Cc cc

We can separate them because they are assorted independently.

For offspring with genotype AABBcc, probability will be:

P(AA) = 1/4

P(BB) = 1/4

P(cc) = 1/4

As all three probabilities has to happen at the same time, it is a "E" rule:

P(AABBcc) =
`((1)/(4)) ((1)/(4)) ((1)/(4))`

P(AABBcc) = 1/64

Probability for the individual to be AABBcc is 1/64 or 1.56%.

Genotype AaBBcc:

P(Aa) = 2/4 = 1/2

P(BB) = 1/4

P(cc) = 1/4

P(AaBBcc) =
`((1)/(2)) ((1)/(4)) ((1)/(4))`

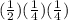
P(AaBBcc) = 1/32

Probability for the individual to be AaBBcc is 1/32 or 3.12%

Genotype AaBbCc:

P(Aa) = 1/2

P(Bb) = 1/2

P(Cc) = 1/2

P(AaBbCc) =
`((1)/(2)) ((1)/(2)) ((1)/(2))`

P(AaBbCc) = 1/8

Probability for the individual to be AaBbCc is 1/8 or 12.5%.