Answer:
The correct answer is B.
Explanation:
Any point can be represented in polar form by using the notation:
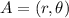 (1)
(1)
And any polar function must satisfy the following definition:

Let
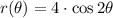 , where
, where
 is the radial distance of any point of the curve from origin and
is the radial distance of any point of the curve from origin and
 is the direction of any point of the curve with respect to +x semiaxis, measured in radians.
is the direction of any point of the curve with respect to +x semiaxis, measured in radians.
Finally, we proceed to graph the function with the help of a graphing tool and attach the result.
Besides, we must remember that cosine is a bounded function, which means that domain and range in rectangular coordinates are represented by:
![Dom\{f\} = [-4,4]](https://img.qammunity.org/2022/formulas/mathematics/high-school/968rsq6znb4o37lbebl5768bue70aidako.png) ,
,
![Ran\{f\} = [-4,4]](https://img.qammunity.org/2022/formulas/mathematics/high-school/v3h71pvmo2ee7mrmxpkjhtm0rz6tlq3jy9.png)
Then, the correct answer is B.