Answer:
base = 519.62, height = 173.21 m
Explanation:
Let the base and height of the triangle be represented by b and h respectively.
Thus,
b = 3h
Area of a triangle =
 x base x height
x base x height
For the given triangle,
area =
 x b x 3h
x b x 3h
=
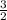 bh
bh
Area of the triangle =
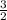 bh
bh
To determine the number of hectares,
36 per hectare = 486
hectare =
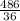
= 13.5
numbers of hectares = 13.5
Area of the hectares = number of hectares x 10 000 m²
= 13.5 x 10 000
= 135000
Total area of the hectares = 135 000 m²
So that,
area of the hectares = area of the triangle
area of the triangle =
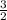 bh
bh
135 000 =
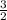 bh
bh
270000 = 3bh
bh =
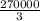
= 90000
bh = 90000
But, b = 3h
3h x h = 90000
3
 = 90000
= 90000
 =
=
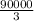
= 30000
h =
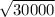
= 173.2051
h = 173.21 m
So that,
b = 3 x 173.2051
= 519.6153
b = 519.62
Therefore, the base of the triangle is 519.62 m, while the height is 173.21 m.