Question

Explain why the graph of quadratic function could not contain both a minimum vertex and a maximum vertex at the same time.

Your explanation should be 3-4 sentences and include at least 6 of the following words/phrase.
-parabola
- u-shaped graph
- vertex
- minimum
- maximum
- y-value of the vertex
- x-value of the vertex
- quadratic function

Answer 1

A quadratic function is a function of the form
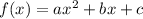
`f(x)=ax^2+bx+c`. The vertex,
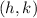
`(h,k)` of a quadratic function is determined by the formula:
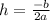
`h= (-b)/(2a)` and
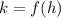
`k=f(h)`; where
`h` is the x-coordinate of the vertex and
`k` is the y-coordinate of the vertex. The value of
`a` determines if the parabola opens upward or downward; if
`a` is positive, the parabola opens upward and the vertex is the minimum value, but if
`a` is negative the graph opens downward and the vertex is the maximum value. Since the quadratic function only has one vertex, it could not contain both a minimum vertex and a maximum vertex at the same time.