Question

How much of a 14% iodine solution should be added to 89 ounces of a 47% iodine solution to get a 29% solution

Answer 1

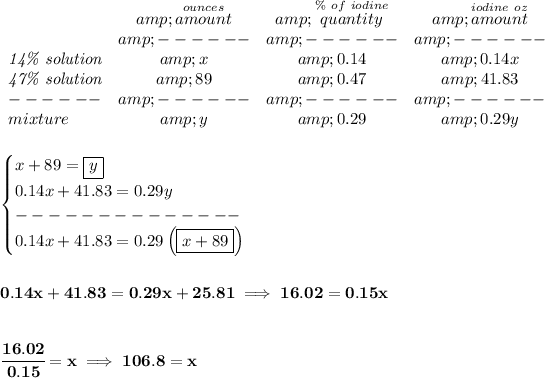
whatever% of anything is just (whatever/100) * anything.

x = ounces of 14% iodine.

y = ounces of 29% iodine.

we know that in a 14% iodine solution, 14% is iodine and the rest is something else, we also know that "x" ounces of the solution have 14% of iodine, how much is that? (14/100) * x, or 0.14x.

likewise, in the 89 ounces of 47% solution there is (47/100) * 89 of iodine.

and likewise as well, in "y" ounces of 29% iodine there is (29/100) * y, or 0.29y of iodine.

bearing in mind that, whatever "x" and "y" may be, x + 89 = y, and that the sum of the iodine amounts also will equate 0.29y.


`\bf \begin{array}{lccclll} &\stackrel{ounces}{amount}&\stackrel{\%~of~iodine}{quantity}&\stackrel{iodine~oz}{amount}\\ &------&------&------\\ \textit{14\% solution}&x&0.14&0.14x\\ \textit{47\% solution}&89&0.47&41.83\\ ------&------&------&------\\ mixture&y&0.29&0.29y \end{array} \\\\\\ \begin{cases} x+89=\boxed{y}\\ 0.14x+41.83=0.29y\\ --------------\\ 0.14x+41.83=0.29\left( \boxed{x+89} \right) \end{cases} \\\\\\ 0.14x+41.83=0.29x+25.81\implies 16.02=0.15x \\\\\\ \cfrac{16.02}{0.15}=x\implies 106.8=x`