Question

Identify this conic section. x 2 - 4x + y 2 - 4y + 4 = 12 line circle ellipse parabola hyperbola

Answer 1

the coefficients for the x² and the y² is the same, with the same sign, namely is +1 for both, and when that happens, what we have is a circle.

Answer 2

Answer: The answer is (B) circle.

Step-by-step explanation: The given equation of the conic section is

`x^2-4x+y^2-4y+4=12.`

We are to select the correct type of the conic section from the given options.

Let us try to find the standard form of the given conic section as follows:

`x^2-4x+y^2-4y+4=12\\\\\Rightarrow (x^2-4x+4)+(y^2-4y+4)=12+4\\\\\Rightarrow (x-2)^2+(y-2)^2=16\\\\\Rightarrow (x-2)^2+(y-2)^2=4^2.`

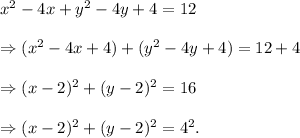
Therefore, the given conic section is a circle with centre (2, 2) and radius 4 units.

The image of the circle is shown in the attached figure.

Thus, (b) Circle is the correct option.