Question

In ΔNOP, n = 910 inches, o = 110 inches and p=820 inches. Find the measure of ∠P to the nearest degree.

Answer 1

Given:

In ΔNOP, n = 910 inches, o = 110 inches and p=820 inches.

To find:

The measure of ∠P to the nearest degree.

Solution:

According to the law of cosine:

`\cos A=(b^2+c^2-a^2)/(2bc)`

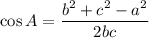
Using law of cosine in triangle NOP, we get

`\cos P=(n^2+o^2-p^2)/(2no)`

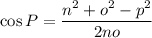
Putting the given values, we get

`\cos P=((910)^2+(110)^2-(820)^2)/(2(910)(110))`

`\cos P=(828100+12100-672400)/(200200)`

`\cos P=(167800)/(200200)`

`\cos P=0.83816`

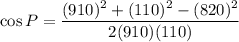
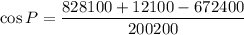
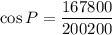
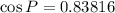
Taking cos inverse on both sides, we get

`P=\cos^(-1)(0.83816)`

`P=(33.05367)^\circ`

`P\approx 33^\circ`

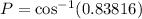
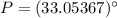
Therefore, the measure of ∠P is 33°.