The electrostatic force between two charges is given by
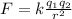
where
k is the Coulomb's constant
q1 and q2 are the two charges
r is their separation
In this problem, the two charges are identical, so we can call them q1=q2=q, and the formula becomes

Since we know the magnitude of the force and the separation between the two charges, we can re-arrange the equation to find the value of each charge:
